Tuesday, July 31, 2007
Wednesday, July 25, 2007
Thierry Brunet Mathematical Poetry Links
This page is reserved for all of Thierry Brunet's Mathematical Poems
No Boundaries
Philosophic Cocktails
Rhesus
Japanese Elvis
Toaster
Posted by
Kaz Maslanka
at
10:31 PM
0
comments
![]()
Labels: Thierry Brunet
A Math Art Moment #5
Posted by
Kaz Maslanka
at
10:22 PM
2
comments
![]()
Labels: math art moment, metaphor
RHESUS By Thierry Brunet
I have noticed something that I find interesting about Thierry's technique when approaching an orthogonal space poem. It seems he acquires three ideas that can be viewed as being near synonymous. He contextualizes them in an orthogonal space which seems to me to work very well together. If one remembers the prismatic structures in my verbogeometry paper then you may also remember that synonyms are viewed existing on a plane. The arrangement of the synonyms on that plane could easily be viewed orthogonally. Furthermore, I think this is why Thierry’s technique works so well.
Posted by
Kaz Maslanka
at
12:06 AM
0
comments
![]()
Labels: orthogonal space poem, Thierry Brunet
Sunday, July 22, 2007
L'anxieux
Posted by
Kaz Maslanka
at
10:30 PM
0
comments
![]()
Labels: Expanded Similar Triangles Poem, French
Japanese Elvis by Thierry Brunet
Posted by
Kaz Maslanka
at
1:46 AM
0
comments
![]()
Labels: Elvis, orthogonal space poem, Thierry Brunet
Friday, July 20, 2007
Foul Of Pray
Posted by
Kaz Maslanka
at
11:15 PM
0
comments
![]()
Labels: Expanded Similar Triangles Poem, politics, war
Thursday, July 19, 2007
The Orthogonal Space Poem

The orthogonal space poem is one of the simplest mathematical structures one can use for mathematical poetry. The structure can be seen in numerous contexts in the discipline of the sciences. Examples in physics would include Newton’s second law “F = ma”, Ohms Law “E = IR”, the kinematical properties of “d = vt”, “p=mv” and E = Fd. Please notice all of the equations are in the form of ‘a’ equals ‘b’ multiplied by ‘c’ or “a = (b)(c)”. This wonderful equation states that the value of one particular concept is equal to the product of two values held by two other concepts. When this equation is depicted in a Cartesian coordinate system you can see that the latter two concepts exist in an orthogonal or perpendicular space.
Before I explain the “orthogonal space poem’s” use in mathematical poetry, let us look at this same mathematical structure in the context of science. Furthermore, before we look at a scientific example let is review a little mathematics. Let us first review the Cartesian coordinate system and its nomenclature.
When we look at the two-dimensional axis of a Cartesian coordinate system, we can see that by randomly picking a point somewhere on the Cartesian plane, then we see there exists is a relationship between this ‘chosen’ point and the point defining the origin of the coordinate system. This relationship is understood by the nomenclature of the coordinate pair (x,y) where x and y are distances along each axis from the origin. Furthermore, if we draw lines from a newly created point, orthogonally (perpendicular) to both the x-axis and the y-axis and taking into consideration the axis system in the background then we will make a rectangle.
The area of a rectangle is product of the lengths of its sides furthermore, in the upcoming example, it is the product of the values for the x and y coordinates of this “chosen” point. I assigned one corner of the rectangle at the origin point to make our example easier to see. Example: Let us pick a point defined by the x-y coordinates of (11,13) and draw lines perpendicular to the axes to illuminate the concept that I just stated. The area of any rectangle is equal to its height multiplied by its base (The product of the lengths of its sides). We have a green rectangle delineated on our axis system. (See figure. 1) The height of our rectangle is 13 units and its base is 11 units. The area of our rectangle is 143 square units … or 13 X 11 = 143 This later example is one of pure mathematics. However if we want to use math as a language then we will have to apply concepts or words to our axis system.
Let us look at a typical physics problem of distance, velocity and time displayed on a two dimensional axis system. Let us assign the y-axis to be levels of velocity in units of miles per hour and the x-axis to be amounts of time in units of hours. Furthermore, let us look at an example using the concept of the “distance an object has traveled is equal to the velocity of that object multiplied by the time the object has traveled.” or “d = vt” In this example let us look at the Cartesian coordinate system as well as its orthogonal construction.
We will use the same pure mathematical example as before but by our contextualizing the axis and assigning the y-axis to represent velocity and the x-axis to represent time, our original point from the last example (11,13) has a new meaning. To reiterate … the point before was in the realm of pure mathematics but now the point represents a moment in time of a speeding object. The object is traveling 13 miles per hour and has been traveling for 11 hours. So to calculate, (d = vt), the distance the object has traveled we must multiply the velocity by the time or 13 miles per hour times 11 hours which equals 143 miles.

In essence, what we have done has been to assign a concept by using words (velocity and time) to our axis system. Moving our attention up or down on the y-axis displays different values of velocity. Moving left and right on, the x-axis displays different amounts of duration or time. Physical experimentation can easily verify the veracity of this equation. In addition, the same experimentation verifies the verbal concepts and their relationship to each other that we have assigned to our axis system. We can see the relationship between the concepts of distance, velocity and time spread out on a two-dimensional plane via our axis system. It is important to note that these concepts occupy orthogonal spaces as well as all equations in the form of a = (b)(c).
The orthogonal space poem possesses the exact same form as our scientific equations however, our intention is poetic as opposed to science.
For our example lets look at the following orthogonal space poem which is titled “Prometheus’s Epistle To Job”
In this poem Prometheus expresses to Job that the suffering of pious people is equal to the arrogance of their God divided by the level of ostentatious generosity imparted by their God.
Lets see how this poem relates to an orthogonal space using a Cartesian coordinate system. We can see how it follows the same structure as the previous physics example.

Another important aspect of creating a orthogonal space poem is to examine all the syntactically different synonymous permutations. Let me reiterate, the structure for an orthogonal poem is a = b c which means that we can solve the equation three different ways. a = b c, b = a/c and c = a/b. When creating an orthogonal space poem you would want to solve and analyze your poem all three different ways to make sure that the poem makes ‘aesthetic sense’ in each different form. Lets look at the latter poem as an example.
The suffering of pious people = The arrogance of their God “divided by” The ostentatious generosity of their God
This poem should also make sense in its two other syntactically different however, synonymous structures.
The arrogance of their God = The suffering of pious people “multiplied by” The ostentatious generosity of their God
And:
The ostentatious generosity of their God = The arrogance of their God "divided by" The suffering of pious people
Posted by
Kaz Maslanka
at
10:12 PM
4
comments
![]()
Labels: God, Job, orthogonal space poem, prometheus
Thursday, July 12, 2007
Toaster By Thierry Brunet
The following is a similar triangles poem sent to me by the French Poet Thierry Brunet.
Posted by
Kaz Maslanka
at
9:51 PM
6
comments
![]()
Labels: proportional poems, Thierry Brunet
Wednesday, July 11, 2007
Physics
Below is another similar triangles poem
Posted by
Kaz Maslanka
at
10:51 PM
0
comments
![]()
Labels: physics, similar triangles poems, Taxonomy
Taxonomy Of Experience
Equations from physics are a taxonomy of correlated experiences.
Posted by
Kaz Maslanka
at
8:49 AM
0
comments
![]()
Monday, July 09, 2007
Korean Dog Dream In Spain
Posted by
Kaz Maslanka
at
12:26 PM
3
comments
![]()
Labels: Bridges, Dog Dream, Korea, orthogonal space poem
Friday, June 29, 2007
The Expanded Similar Triangles Poem
The similar triangles method for constructing mathematical poems easily enables us to manipulate a logical structure for metaphoric expression. What this pedagogical blog entry is trying to accomplish is the introduction and showing of the expanded version of the similar triangles poem. This poetic structure is logically more complex however, still easy to manipulate.
Before you read further, please be familiar with the similar triangles poem. You may read about it here.
The 'regular' similar triangles poem only utilizes two similar triangles and is defined as an expression of two ratios. The expanded similar triangles poem utilizes ‘n’ number of ratios and is able to create very large (infinite) complex mathematical poems and still have a logical structure to act as a source domain for our metaphoric expression.
I am going to create an expanded similar triangle poem made of three similar triangles to serve as our example.
The following image shows three similar triangles with three different sets of relationships:‘a is to b’ as ‘c is to d’ and ‘e is to f’
 The trick in the construction of the poem is to add or subtract the numerators of two of the relationships and also add or subtract the two corresponding denominators of the same two relationships. The next image shows us how we construct our three ratios and associated them differently with subtraction and addition to form six flavors. There are actually 12 flavors possible however; I wish to focus on these six, which I consider the best ones to give us a good understanding of what is occurring in this method.
The trick in the construction of the poem is to add or subtract the numerators of two of the relationships and also add or subtract the two corresponding denominators of the same two relationships. The next image shows us how we construct our three ratios and associated them differently with subtraction and addition to form six flavors. There are actually 12 flavors possible however; I wish to focus on these six, which I consider the best ones to give us a good understanding of what is occurring in this method.
Each flavor yields a group of six equations after the flavor is solved for each variable. The next six images show the six syntactical arrangements for each flavor. There are at least seventy-two different syntactical arrangements however; I wish to focus on what I feel to be the thirty-six most important ones. The next six images show each of the six groups created by each flavor.
FLAVOR ONE YIELDS: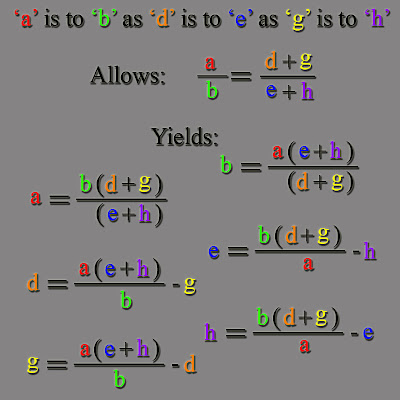 FLAVOR TWO YIELDS:
FLAVOR TWO YIELDS: FLAVOR THREE YIELDS:
FLAVOR THREE YIELDS: FLAVOR FOUR YIELDS:
FLAVOR FOUR YIELDS: FLAVOR FIVE YIELDS:
FLAVOR FIVE YIELDS: FLAVOR SIX YIELDS:
FLAVOR SIX YIELDS: Lets now create a poem using the following text.
Lets now create a poem using the following text.
a = Love Lies
b = The Lonely
d = Sugar
e = The Starving
g = Sexual Conquests
h = The Insecure
The structure follows as thus: ‘Love Lies’ is to ‘The Lonely’ as ‘Sugar’ is to ‘The Starving’ as ‘Sexual Conquests’ are to ‘The Insecure’
We then substitute the variables with the poetic phrases to yield thirty-six poems in six groups relating back to the flavors shown above.
I find it interesting to savor each syntactical permutation to get a ‘feel’ for each poetic expression.
GROUP ONE CONSTRUCTED FROM FLAVOR ONE: GROUP TWO CONSTRUCTED FROM FLAVOR TWO:
GROUP TWO CONSTRUCTED FROM FLAVOR TWO: GROUP THREE CONSTRUCTED FROM FLAVOR THREE:
GROUP THREE CONSTRUCTED FROM FLAVOR THREE: GROUP FOUR CONSTRUCTED FROM FLAVOR FOUR:
GROUP FOUR CONSTRUCTED FROM FLAVOR FOUR: GROUP FIVE CONSTRUCTED FROM FLAVOR FIVE:
GROUP FIVE CONSTRUCTED FROM FLAVOR FIVE: GROUP SIX CONSTRUCTED FROM FLAVOR SIX:
GROUP SIX CONSTRUCTED FROM FLAVOR SIX:
Many of the links below are examples of the expanded similar triangles poem.
Posted by
Kaz Maslanka
at
8:23 PM
2
comments
![]()
Labels: Expanded Similar Triangles Poem, similar triangles poems
Love And Ego
Posted by
Kaz Maslanka
at
8:02 PM
0
comments
![]()
Wednesday, June 27, 2007
Another From Gregory Vincent St. Thomasino

The NYC philosopher/poet Gregory Vincent St. Thomasino would like to see a math poem of his cartoon shown above. The result is a similar triangles poem shown below.

Posted by
Kaz Maslanka
at
11:59 PM
0
comments
![]()
Labels: Gregory Vincent St. Thomasino, similar triangles poems
Sunday, June 24, 2007
Two Emblem Poems From Thierry Brunet
I just received two images from Thierry Brunet and would like to discuss them. Both of these images I would consider emblem poems with a mathematical emphasis. Thierry has made some interesting connections in these poems. The first one I wish to point out is in his piece titled "HeXaedron". A Hexahedron is commonly known as a cube as well as being a regular polyhedral. Thierry asks “how many platonic solids do we need to dream TOMORROW? The answer depends on many things but my first thought is the question of what dimensions are we limited to? There are 5 platonic solids in the third dimension 6 in the fourth dimension and 3 in every dimension above the fifth. So if we include all dimensions we have an infinite choice. However, he is pointing at time by mentioning the PRESENT as well as TOMORROW so this also makes me entertain the idea of relativity.
Another thing I find interesting is the statement “Don’t forget the empirical BEAUTY of experience. Thierry now connects by capital letters time with beauty however, more important to me is the idea of an aesthetic of beauty tied directly to logic through an empirical process. Some artists would argue that beauty cannot be expressed with a logical statement. I feel they should be nauseous from eating only the icing and none of the cake.
The second piece is titled “mouTH THeory” I like this piece as well because I find my reality can be seen in terms of topology and beliefs are the forces that distort that topology. And here we have a lady whose scream swallows it.
Posted by
Kaz Maslanka
at
11:35 PM
0
comments
![]()
Labels: emblem poems, Thierry Brunet
Saturday, June 16, 2007
Two Mathematical Poems For Gregory Vincent St. Thomasino
“Profundity” is the title of the first mathematical poem and the second “Logoclasody”.
 The drawing above is by Gregory Vincent St. Thomasino
The drawing above is by Gregory Vincent St. Thomasino
If discourse is a river then what is a lake?
Is not the Philosopher a dam?
Logoclasody
The two mathpoems above are similar triangles poems
Also related is the Avrin proposition
Posted by
Kaz Maslanka
at
10:45 AM
0
comments
![]()
Labels: Avrin proposition, Gregory Vincent St. Thomasino, Logoclasody, similar triangles poems
Friday, June 01, 2007
The Account Of Oscar
The following is a mathematical adaptation and edition of an Oscar Wilde quote.
Posted by
Kaz Maslanka
at
7:09 PM
0
comments
![]()
Labels: orthogonal space poem, Oscar Wilde
Thursday, May 31, 2007
Fall Well
I forgot to post this two weeks ago.
Posted by
Kaz Maslanka
at
9:53 PM
0
comments
![]()
Labels: Jerry Falwell, orthogonal space poem
Wednesday, May 30, 2007
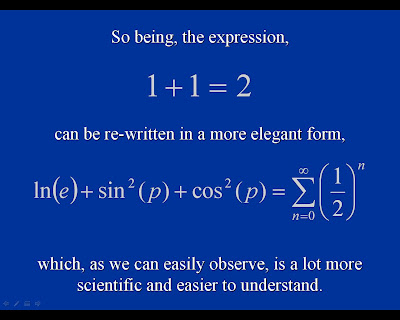
Duchampian Identities?
The following slides came to me anonymously however; I would have loved to credit the author if given the chance. The original intent of these slides was humor but on a deeper level this cerebral dance reminds me of the artistic equivalent of Duchamp’s found objects. Here our author has found bits and pieces of historically significant mathematical identities whose purpose are totally unrelated to the context of this wonderful buffoonery. He/she has logically pieced them together to take your mind on a trip through a kaleidoscopic mathematical collage logically woven together to end full circle. I love it … so enjoy! And thanks to whoever created it.
Posted by
Kaz Maslanka
at
6:19 PM
3
comments
![]()
Labels: Duchamp, Mathematical Identities